#quadratic equations visual method

[9nid]

🧠 Master Graphical Applications of Quadratic Equations – Part 2 | JEE Series Part-15 | By 9nid

🎯 Introduction Quadratic Equations are one of the most tested and important topics in JEE Mathematics, and understanding their graphical behavior can make even the toughest questions feel easy! In Part-15 of the JEE Series by @9nid, we dive deeper into the Graphical Application of Quadratic Equations (Part-2) — focused on visualizing inequalities and interpreting expressions using…

[edusquaremaths]

🧠 Master Graphical Applications of Quadratic Equations – Part 2 | JEE Series Part-15 | By 9nid

🎯 Introduction Quadratic Equations are one of the most tested and important topics in JEE Mathematics, and understanding their graphical behavior can make even the toughest questions feel easy! In Part-15 of the JEE Series by @9nid, we dive deeper into the Graphical Application of Quadratic Equations (Part-2) — focused on visualizing inequalities and interpreting expressions using…

[imgvai127]

Futuristic Math Lab

inside a futuristic 3d mathematics lab with white walls and soft blue-white neon lighting, display a glowing quadratic equation above the central lab table, the expression visually transforms into two separate methods: on the left side, the equation splits into binomial factors (ax + b)(cx + d); on the right side, it rearranges into a perfect square form (x + p)² = q, each method animates step-by-step with minimal number cues, and solution points appear on a coordinate grid below as glowing intersections with the x-axis, behind the table, the smartboard shows a clean holographic comparison of both methods side by side using visual motion only, no people in the scene, camera angle: slightly elevated diagonal view showing both solving paths, the x-intercepts, and the smartboard clearly.

[imgvai134]

Futuristic Math Lab

inside a futuristic 3d mathematics lab with white walls and soft blue-white neon lighting, display a glowing quadratic equation above the central lab table, the expression visually transforms into two separate methods: on the left side, the equation splits into binomial factors (ax + b)(cx + d); on the right side, it rearranges into a perfect square form (x + p)² = q, each method animates step-by-step with minimal number cues, and solution points appear on a coordinate grid below as glowing intersections with the x-axis, behind the table, the smartboard shows a clean holographic comparison of both methods side by side using visual motion only, no people in the scene, camera angle: slightly elevated diagonal view showing both solving paths, the x-intercepts, and the smartboard clearly.

[imgvai2]

Futuristic Math Lab

inside a futuristic 3d mathematics lab with white walls and soft blue-white neon lighting, display a glowing quadratic equation above the central lab table, the expression visually transforms into two separate methods: on the left side, the equation splits into binomial factors (ax + b)(cx + d); on the right side, it rearranges into a perfect square form (x + p)² = q, each method animates step-by-step with minimal number cues, and solution points appear on a coordinate grid below as glowing intersections with the x-axis, behind the table, the smartboard shows a clean holographic comparison of both methods side by side using visual motion only, no people in the scene, camera angle: slightly elevated diagonal view showing both solving paths, the x-intercepts, and the smartboard clearly.

[imgvai82]

Futuristic Math Lab

inside a futuristic 3d mathematics lab with white walls and soft blue-white neon lighting, display a glowing quadratic equation above the central lab table, the expression visually transforms into two separate methods: on the left side, the equation splits into binomial factors (ax + b)(cx + d); on the right side, it rearranges into a perfect square form (x + p)² = q, each method animates step-by-step with minimal number cues, and solution points appear on a coordinate grid below as glowing intersections with the x-axis, behind the table, the smartboard shows a clean holographic comparison of both methods side by side using visual motion only, no people in the scene, camera angle: slightly elevated diagonal view showing both solving paths, the x-intercepts, and the smartboard clearly.

[imgvai143]

Futuristic Math Lab

inside a futuristic 3d mathematics lab with white walls and soft blue-white neon lighting, display a glowing quadratic equation above the central lab table, the expression visually transforms into two separate methods: on the left side, the equation splits into binomial factors (ax + b)(cx + d); on the right side, it rearranges into a perfect square form (x + p)² = q, each method animates step-by-step with minimal number cues, and solution points appear on a coordinate grid below as glowing intersections with the x-axis, behind the table, the smartboard shows a clean holographic comparison of both methods side by side using visual motion only, no people in the scene, camera angle: slightly elevated diagonal view showing both solving paths, the x-intercepts, and the smartboard clearly.

[imgvai80]

Futuristic Math Lab

inside a futuristic 3d mathematics lab with white walls and soft blue-white neon lighting, display a glowing quadratic equation above the central lab table, the expression visually transforms into two separate methods: on the left side, the equation splits into binomial factors (ax + b)(cx + d); on the right side, it rearranges into a perfect square form (x + p)² = q, each method animates step-by-step with minimal number cues, and solution points appear on a coordinate grid below as glowing intersections with the x-axis, behind the table, the smartboard shows a clean holographic comparison of both methods side by side using visual motion only, no people in the scene, camera angle: slightly elevated diagonal view showing both solving paths, the x-intercepts, and the smartboard clearly.

[cecestudies]

Essential Topics You Must Master for A Level Maths Success

Struggling with A Level Maths revision? In this guide by Exam Tips, we break down the essential topics you must master for A Level Maths success. Whether you're aiming for an A or just trying to pass confidently, focusing on the right areas can make all the difference. From core algebra and calculus to statistics and mechanics, we highlight the key concepts that appear frequently in exams and how to tackle them efficiently. With smart strategies and topic-focused revision, you’ll boost your confidence and performance. Let Exam Tips be your go-to source for structured A Level Maths revision, practice insights, and proven study methods. Start mastering the topics that matter most—and leave the guesswork behind.

Mastering Algebraic Techniques and Expressions

Algebra forms the backbone of most questions in A Level Maths. You must be comfortable simplifying expressions, solving equations, and manipulating algebraic fractions. Factoring, expanding brackets, and completing the square are all skills that appear across different types of problems. Understanding how to use functions and their notations is equally important, especially when combined with graph sketching and transformations. Exam Tips recommends starting your A Level Maths revision by reinforcing these core algebraic techniques since they often build into more complex topics like calculus and trigonometry.

Deep Understanding of Calculus Fundamentals

Calculus is one of the most heavily weighted topics in A Level Maths exams. You’ll need to grasp the principles of differentiation and integration, including their real-world applications. This includes curve sketching, finding stationary points, calculating areas under curves, and solving problems involving rates of change. It's crucial to not only memorise formulas but also understand when and how to apply them effectively. A structured approach from Exam Tips ensures that your A Level Maths revision includes progressive problem-solving that reinforces both basic and advanced calculus skills.

Trigonometry and Its Real-World Applications

Trigonometry plays a key role in both pure and applied mathematics. You should be confident in using identities, solving equations, and working with radian measures. Real-world applications like modelling wave functions and solving vector problems often incorporate trigonometric principles. As you progress in your A Level Maths revision, practicing with unit circle diagrams and trigonometric transformations will help solidify your understanding. Exam Tips suggests integrating these concepts with questions from mechanics and coordinate geometry to deepen your problem-solving ability.

Working with Functions, Graphs, and Transformations

A thorough understanding of functions and graphs is essential to A Level Maths success. You’ll encounter a variety of functions including linear, quadratic, exponential, and logarithmic forms. Being able to draw, transform, and interpret graphs is a skill tested across both Paper 1 and Paper 2. Students are often required to analyse roots, intercepts, and asymptotes. As part of your A Level Maths revision plan, Exam Tips encourages consistent graph-based practice to develop a visual intuition for mathematical relationships and behaviour of functions.

Statistical Techniques and Probability Concepts

The statistics component in A Level Maths includes data representation, hypothesis testing, and interpreting statistical diagrams. It also requires understanding of key distributions, such as binomial and normal. Probability questions often involve Venn diagrams, conditional probability, and expected outcomes. These questions test your ability to apply logical thinking to real-world scenarios. Exam Tips recommends integrating statistical problem sets into your A Level Maths revision early on, so you become comfortable with data interpretation and numerical reasoning.

Mechanics: Kinematics and Newton’s Laws

Mechanics introduces physical applications of mathematical theory, with a focus on motion, forces, and energy. You’ll need to understand velocity-time graphs, equations of motion, and the principles of Newton’s laws. Problems often involve modelling real-life scenarios with diagrams and resolving forces in components. Exam Tips advises students to connect mechanics with algebra and calculus topics, as many problems overlap conceptually. A Level Maths revision should include both conceptual learning and practice-based assessments in this area.

Solving Realistic Modelling and Word Problems

A Level Maths increasingly includes worded problems that require mathematical modelling. These questions assess not only your computational ability but also your analytical thinking. You must identify relevant information, translate it into mathematical form, solve the problem, and interpret your result. Topics such as exponential growth and decay, optimisation, and dynamics often appear in this format. As you revise with Exam Tips resources, focus on breaking down complex questions into manageable parts. Developing this skill will greatly improve your overall exam performance.

Conclusion

Succeeding in A Level Maths requires more than just learning formulas — it demands deep understanding and consistent practice. By focusing your A Level Maths revision on the essential topics discussed above, you’ll build a strong, exam-ready foundation. Exam Tips is committed to helping students unlock their potential with focused guidance, practical resources, and expert strategies. Remember, success in maths isn’t about doing everything — it’s about doing the right things the right way. Stay focused, stay curious, and trust the process.

[sathcreation]

Expert IIT Mathematics Teacher at Gritty Tech

Introduction to IIT JEE Mathematics Excellence

The journey to cracking the prestigious IIT JEE begins with mastering one of its most challenging components – Mathematics. At Gritty Tech, we understand the intensity and depth required to succeed in this subject. That's why we offer specialized coaching under the guidance of expert IIT Mathematics teachers who not only know the syllabus but also understand the psychology and strategy behind high-stakes exams For More…

Our teaching is not just about covering topics. It’s about building a solid mathematical foundation, developing analytical thinking, and fostering a problem-solving mindset.

Why Expert Teachers Make a Difference

IIT JEE Mathematics is not about rote learning. It demands creativity, speed, accuracy, and deep conceptual understanding. An expert IIT Maths teacher brings all these elements to the table. At Gritty Tech, our instructors are selected through a rigorous process. Each of them has years of experience in mentoring toppers, with proven results across multiple batches.

Key Attributes of Our Expert Maths Faculty:

In-depth Subject Knowledge: Every teacher at Gritty Tech has a mastery over the entire JEE syllabus including Algebra, Calculus, Coordinate Geometry, Trigonometry, Probability, and more.

Strategic Teaching Methods: They know how to break down complex problems into simpler steps and highlight shortcuts that save time in exams.

Focus on Conceptual Clarity: Instead of encouraging memorization, our approach ensures students understand the 'why' behind every formula.

Real Exam Insight: Teachers share insights from previous year papers, trends, and changing patterns in JEE to keep students one step ahead.

Individual Mentorship: Every student is different. Our experts provide personal guidance to overcome weaknesses and build on strengths.

A Curriculum Built for Success

At Gritty Tech, the Mathematics curriculum is not generic. It is crafted specifically for IIT JEE aspirants, keeping in mind the latest trends, difficulty levels, and expected question formats. Our curriculum is reviewed and refined every year by a panel of experts.

The Core Topics Covered Include:

Algebra: Quadratic equations, Complex numbers, Matrices & Determinants, Permutations & Combinations

Calculus: Limits, Continuity, Differentiability, Application of Derivatives, Integrals, Differential Equations

Coordinate Geometry: Straight lines, Circles, Conic Sections

Trigonometry: Identities, Equations, Inverse Trigonometric Functions

Vectors and 3D Geometry

Probability and Statistics

Each topic is taught from the ground up, beginning with theory and moving into solved examples, practice sheets, test series, and revision strategies.

Teaching Methodology at Gritty Tech

What truly sets Gritty Tech apart is not just the content we teach, but how we teach it.

Interactive Concept Classes

Our sessions are designed to be interactive. Teachers use smart boards, real-life analogies, and visual aids to explain abstract mathematical ideas. Every class ends with a set of practice problems that help reinforce the day’s learning.

Doubt Resolution in Real-Time

Students never have to wait till the end of the week to clear their doubts. With dedicated doubt-solving sessions and availability of the faculty even outside class hours, learning continues seamlessly.

Regular Testing and Feedback

Frequent quizzes, weekly tests, and full-length mock exams are conducted to ensure students are exam-ready. After each test, teachers provide personalized feedback and performance analysis.

Adaptive Learning

Not every student learns the same way. Our experts use adaptive learning techniques to identify the learning style of each student and modify their approach accordingly.

[speedywonderlandtrash]

Class 9 Math Annual Exam with Model 3

Class 9 Math Annual Exam with Model 3

  Class 9 math exam preparation guide,last minute math exam tips for Class 9,Class 9 math practice questions and solutions,model 3 math prep for Class 9 exam,effective study techniques for Class 9 math exam Math Time: 3                              Hours Class: 9                     Total Marks: 100 Section A: Objective (25 Marks) Multiple Choice Questions: (Write the correct answer on the answer sheet) 1 × 15 = 15 1. a, ar, ar², ar³ is which type of sequence? (a) Geometric (b) Arithmetic (c) Infinite (d) Constant 2. If 7x + 2, 5x + 12, 2x - 1 form an arithmetic progression, what is the value of x? (a) -23 (b) 23 (c) ±23 (d) 21 3. What is the 15th term of the sequence 4 + 8 + 16 + ........? (a) 65536 (b) 131072 (c) 146384 (d) 32768 4. logb n , what is the argument? (a) k (b) n (c) b (d) log 5. What is the base of lnx? (a) e (b) 10 (c) x (d) y 6. What is logbAx? (a) x (b) A (c) b (d) xlogbA 7. If the sum and difference of the digits of a two-digit number are 10 and 4 respectively, what is the number? (a) 47 (b) 27 (c) 37 (d) 57 8. Which point is on the x-axis? (a) (2, 0) (b) (-3, 5) (c) (0, 3) (d) (-2, -2) 9. For θ = 45° - i. sin2 θ + tan2 θ = ii. sin2 θ + cos2 θ = iii. 1 - sin2 θ = Which of the following is correct? (a) i and ii (b) i and iii (c) ii and iii (d) i, ii, and iii 10. Based on the following information, answer questions 10 and 11: In right-angled triangle ABC, ∠C = β, ∠B = α, AB = 7, BC = 25 cm, and AC = 24 cm. What is the length of the side opposite to angle β? (a) 7

(b) 24 (c) 25 (d) 6 11. For which of the following angles is the length of the adjacent side 24 cm? (a) α (b) β (c) α + β (d) α - β 12. In the first quadrant, how are all trigonometric ratios? (a) Positive (b) Negative (c) 0 (d) Even 13. What is cos 150°? (a) (b) (c) - (d) - 14. How many types of data are there? (a) 2 (b) 3 (c) 4 (d) 5 Class 9 Math Annual Exam with Model 2 15. If ∑fi|xi - Mo| = 216.92 and n = 20, what is the mean deviation calculated from the median? (a) 8×85 (approximately) (b) 10×85 (approximately) (c) 9×85 (approximately) (d) 7×85 (approximately) 16. Write the condition for a, b, c to be in a geometric progression. 17. What is the sum of the first n natural numbers? 18. What is log₂ 16? 19. Write the formula for logb () 20. What is the discriminant of the equation ax² + bx + c = 0? 21. What is the meaning of the word 'Metron'? 22. In the second quadrant, what is the sign of cos θ? 23. What is cot(90° - θ)? 24. What is the range typically represented by? 25. What is the relationship between the mean deviation M.D and the range R for two unequal data sets? 1. Answer the following questions: 2 × 13 = 26 (a) If the third term and fifth term of an arithmetic progression are -12 and 26, respectively, find the first term and common difference. (b) For the series 2 + 4 + 6 + 8 + ..., if the sum of the first n terms is 2550, find the value of n. (c) Find the general term of the arithmetic progression 5, 12, 19, 26, ... (d) If log₅ x = 3, what is the value of x? (e) At a 10% compound interest rate, in how many years will the principal triple? (f) Solve the system of equations using substitution method: 2x + 3y = 32 11y - 9x = 3 (g) Solve the equation 3x² - 2x - 1 = 0 using the quadratic formula. (h) If 12 cot θ = 7, find the value of cos θ. (i) From a point 15 meters away from the base of a tower, the angle of elevation to the top of the tower is 30°. Find the height of the tower. (j) Convert radians to degrees. (k) For the angle θ = ∠XOP in standard position, find the trigonometric ratios for the point A(-4, -3) on the terminal arm. (l) Find the range of the data set: 7, 5, 12, -5, 0, 10. (m) Find the cumulative frequency distribution for the given data: x 60 61 62 63 64 65 66 67 f 2 0 15 30 25 12 11 5 Answer the following descriptive questions (based on the visual context):                                       7 × 7 = 49 2. Consider the following two geometric progressions: (i) x + 1, x + 5, x + 10, ....... (ii) 2 - 4 + 8 - 16 + .... (a) Find the value of x in the first geometric progression x + 1, x + 5, x + 10, ....... (3 marks) (b) Which term of the second geometric progression 2 - 4 + 8 - 16 + ..... is equal to 256? (4 marks) 3. In Arup's school hall, there are 30 rows of benches. The first, second, and third rows have seats in the following quantities: (k + 12), (3k + 10), and (7k + 4) respectively. (a) Find the value of k if the number of seats forms an arithmetic progression. (2 marks) (b) How many seats are there in the last row? (2 marks) (c) Find the total number of seats in the hall. (3 marks) 4. Given that: A = B = , and C = (a) If A = 128, find the value of p. (3 marks) (b) Prove that B ÷ C = . (4 marks) 5. An earthquake is felt in two locations in Bangladesh, Sylhet and Chittagong, on the same day. The magnitude of the earthquake in Sylhet is 6.5, and the earthquake in Chittagong is 17 times stronger. The magnitude of the earthquake in India, which is located near Bangladesh, is 7.1. (a) Find the magnitude of the earthquake in Chittagong. (3 marks) (b) Compare the intensity of the earthquakes in Sylhet and India, and determine which place has a higher risk. (4 marks) 6. Setu's mother bought 25 ducklings and 30 chicks for 5000 taka. If she had bought 20 ducklings and 40 chicks at the same rate, she would have spent 500 taka less. (a) What is the cost of one duckling and one chick? (4 marks) (b) After some time, if each duck is sold for 250 taka and each chicken for 160 taka, what will be her total profit? (3 marks) 7. Samiya bought 4 pens and 2 notebooks for 100 taka from a shop. Lamiya bought 2 pens and 3 notebooks for 110 taka from the same shop at the same price. (a) Form the system of equations from the given information and determine its nature. (3 marks) (b) Find the price of each notebook and pen. (4 marks) 8. Roni and Tahmid were walking along the riverbank when they saw the top of a 100-meter tall tree on the opposite bank. The angle of elevation to the top of the tree from their position was 60°. Later, Tahmid moved back a little and saw that the angle of elevation from his new position was 45°. (a) Calculate the distance from Roni and Tahmid to the opposite bank of the river. (3 marks) (b) How much further back did Tahmid move from Roni? (4 marks) 9. A car travels from Dhaka to Khulna. The rear wheel of the car rotates 12 times per second, and the radius of the wheel is 0.5 meters. The distance from Dhaka to Khulna subtends an angle of 2° at the center of the Earth. (a) How far will the car travel in one full rotation of the wheel? (2 marks) (b) Calculate the speed of the car. (2 marks) (c) How long will it take the car to reach Khulna from Dhaka? (3 marks) 10. The frequency distribution table for the mathematics marks of 125 students of Class 9 is given below: Marks Obtained 10 - 20 20 - 30 30 - 40 40 - 50 50 - 60 60 - 70 Number of Students 10 17 30 40 20 8 (a) What is the average mark of the students in Class 9 in mathematics? (3 marks) (b) Using the assumed mean method or direct method, calculate the deviation from the mean. (4 marks) 11. The following is the frequency distribution table for the number of absences of 40 students in a class last month: Absence Days 1 - 4 5 - 8 9 - 12 13 - 16 17 - 20 Number of Students 5 11 7 2 1 (a) What is the range of the first 12 prime numbers? (2 marks) (b) How many students attended the class every day last month? (2 marks) (c) Calculate the range from the frequency distribution table. (3 marks) Read the full article

[proceduralcaves]

Source notes: Explicit Generation of 3D Models of Solution Caves for Virtual Environments

(Boggus & Crawfis, 2009)

Type: Journal Article

Keywords: Synthetic Environments, Caves, Phreatic Passage, Vadose Passage, and Combination Passage

Relevance: 3/5

The authors endeavour to create virtual caves by understanding and observing how they are formed in reality. Their method produces different 3D models based on two types of cave passages, that can then be combined to form larger systems.

The paper begins by briefly mentioning some computer games (the abstract mentions movies in relation to virtual environments but the researchers' focus seems to be on games), Dig Dug, Tomb Raider and Halo. They proceed to discuss the need for, and merits of, procedural generation of content due to the increasing complexity of 3D environments in games and the power of algorithmic methods, as well as the relative paucity of research on the generation of caves.

A definition of caves is given as "natural voids underground that are large enough for a person to enter". The type of cave being recreated is a solution cave, formed when rock is dissolved by acidic water. A process which can take several thousands of years, and which requires there to be "sufficient groundwater recharge to dissolve rock and enough drainage of the solution to remove sediment from the area". It is solution caves that speleothems (mineral formations) are often found in, a result of precipitation after the formation of the main cave passages. As speleothems are visually interesting, recognisable as cave features and found in most examples of computer game caves, the Masters project and its technical artefact will also focus on solution caves.

Referenced are examples of polygonal models created using spatial data of caves from surveys. Such examples are insufficiently detailed and inflexible for computer games but may be worth referencing to illustrate the challenges of 3D cave generation. The same can be said in terms of the cost and time investment — and again, static nature of — cave models based on spatial data obtained by laser and ultrasound scanning, which (Boggus & Crawfis, 2009) also mention.

Two types of cave passages are described, phreatic and vadose. Phreatic passages are rounded and horizontal in shape, widened in all directions. Vadose are vertical slots formed by rapidly moving and descending water.

The technique used to model these passages involves extruding one Bezier curve (a smooth, continuous curve created using "control points" as parameters to a formula) along the trajectory of another.

For phreatic passages, the following curves are used:

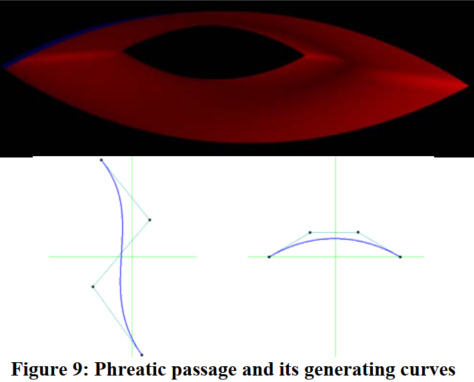
The curve on the right is extruded along the curve on the left (representing the flow of water). The resulting surface is copied and reflected to create both the ceiling and floor of the passage. A quadratic equation is used to create curves of differing heights and widths that can then be extrudede.

Vadose passages are created in a similar manner. The curve on the right is "swept" along the curve on the left to create one wall, before repeating with the trajectory curve reflected. The tops and bottoms of each wall are then connected.

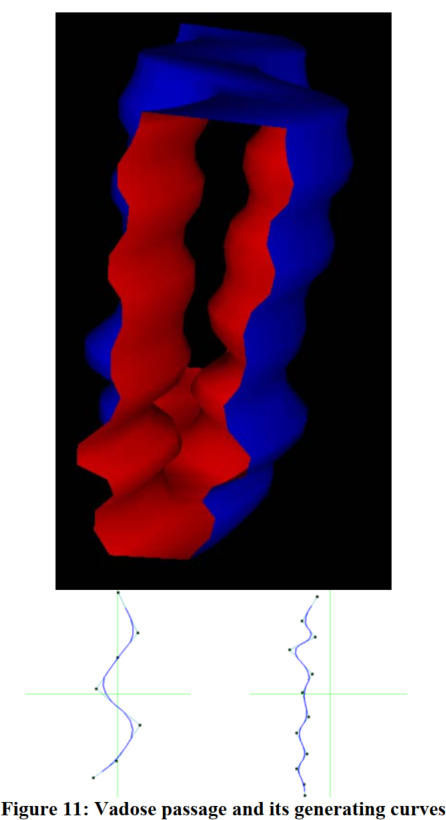
Combination passages are created by sweeping cross sections along trajectory curves or by combining models of separate passages and eliminating clipping vertices and vertices from one passage that are inside the cavity of another.

The paper concludes by presenting textured models and suggesting that cave features and surface details could be created through techniques such as bump mapping and displacement mapping.

The method used is simple and computationally inexpensive. It makes it easy to create varied surfaces and passage shapes. However, a lot of work is required to join passages and create larger systems. There is no detailed information on generating speleothems. Features such as collision detection and texturing are not covered, nor is parameterisation or customisation of the models outside of altering the input curves. It is relevant to include as an example of a basic method for generating virtual caves and because it uses a unique technique.

[shiva10010]

JEE Main 2025: A Guide to the Mathematics Syllabus

An Overview of the Syllabus

The JEE Main Maths syllabus encompasses 14 fundamental units, spanning various branches of mathematics:

Sets, Relations and Functions: This unit lays the foundation with concepts like set theory, operations on sets, relations, functions, and their properties.

Complex Numbers and Quadratic Equations: Dive into the world of complex numbers, including their representation, operations, and applications in solving quadratic equations.

Matrices and Determinants: Explore matrices, their algebra, determinants, properties, and their applications in solving systems of linear equations.

Permutations and Combinations: Master the art of counting and arranging objects with permutations and combinations.

Binomial Theorem and its Simple Applications: Learn about the binomial theorem for expanding powers of a binomial and its applications in various problems.

Sequence and Series: Understand the concept of sequences and series, their operations, convergence, and divergence.

Limit, Continuity and Differentiability: This unit focuses on the fundamental concepts of calculus, including limits, continuity, and differentiability of functions.

Integral Calculus: Explore integration techniques, applying them to solve definite and indefinite integrals and their applications.

Differential Equations: Learn about differential equations, their formation, and solving techniques for various types of equations.

Coordinate Geometry: Delve into the world of coordinates, straight lines, circles, parabolas, hyperbolas, and their properties.

Three-Dimensional Geometry: Visualize and understand lines, planes, spheres, cones, and cylinders in three-dimensional space.

Vector Algebra: Master operations on vectors, their properties, scalar product, vector product, and their applications.

Statistics and Probability: This unit introduces statistical methods for data analysis and probability theory for solving problems involving chance.

Trigonometry: Refresh your knowledge of trigonometric functions, identities, equations, and their applications in solving geometrical problems.

Tips for JEE Main 2025 Maths Preparation

Focus on Concepts: A strong foundation in core concepts is essential. Don't just memorize formulas; strive to understand the underlying principles.

Practice Regularly: Solve problems from various sources, including previous years' JEE Main papers and mock tests.

Clear Your Doubts: Don't hesitate to seek clarification from teachers or mentors for any doubts you encounter.

Time Management: Practice solving problems within the stipulated time frame to develop exam temperament.

Focus on High-Weightage Topics: Analyze previous years' papers to identify topics with a higher weightage and prioritize your preparation accordingly.

By following these tips and diligently studying the syllabus, you can confidently approach the JEE Main 2025 Mathematics section. Remember, consistency, hard work, and a strategic approach are key to success!

[siampiner]

Solving quadratic equations calculator

SOLVING QUADRATIC EQUATIONS CALCULATOR FREE

BYJUS online quadratic equation calculator.

SOLVING QUADRATIC EQUATIONS CALCULATOR FREE

Use ↑ and ↓ to toggle between the roots.īuy a Casio fx-991EX calculator on Amazon using this affiliate link to help support this site. Quadratic Equation Calculator is a free online tool that displays the roots of the given quadratic equation. Open the quadratic equation solve mode: MODE 5 3.Furthermore, this also doesn’t require you to remember the quadratic formula, for exams where you are not provided with the formula, although it’s probably worth remembering the quadratic formula anyway.įor example, I will solve the following equation for x. For equations with real solutions, you can use the graphing tool to visualize the solutions. The Quadratic Formula Calculator finds solutions to quadratic equations with real coefficients. This method of solving equations is much faster than typing in the quadratic formula and doesn’t require you to manually flip the + and − signs in the quadratic formula as ± can’t be entered directly into the calculator. Quadratic Formula Calculator Step 1: Enter the equation you want to solve using the quadratic formula. Need more problem types Try MathPapa Algebra Calculator. This also works with quadratic equations which have no real roots: the roots can be provided as complex numbers. Quadratic equations have an x2 term, and can be rewritten to have the form: a x 2 + b x + c 0. Instead of needing to enter the quadratic formula with a, b and c substituted, you can simply insert a, b and c into the quadratic equation solver and the calculator will calculate the roots for you and display them onscreen as x 1 and x 2. In this case, set 'To value' to 0.Quadratic equations can be solved using the quadratic formula which is stored on the calculator and can be used from the equation mode. To find the roots, set y = 0 and solve the quadratic equation 3x 2 - 12x + 9.5 = 0. 3- To find the y co-ordinate of the vertex, simply plug the value of b 2a b 2 a into the equation for x and solve for y. All quadratic equations can be solved by graphing, unless they happen to. For example, enter the value 0 into cell A2 and repeat steps 5 to 9. Answer: 1- Get the equation in the form of y ax2 +bx +c y a x 2 + b x + c. Calculators can be great time-savers when you're allowed to use them. An algebra calculator that finds the roots to a quadratic equation of the form ax2+bx+c0 a x 2+ b x + c 0 for x x, where ane0 a 0 through. Excel finds the other solution if you start with an x-value closer to x = -1. There are other ways of solving a quadratic. Want to solve quadratic equations quickly Try our advanced free Quadratic Equation Calculator to solve all equations instantly and error-free. Enter values for a, b, c and d and solutions for x will be calculated. This program computes roots of a quadratic equation when coefficients a, b and c are known. Use this calculator to solve polynomial equations with an order of 3 such as ax 3 + bx 2 + cx + d 0 for x including complex solutions. Click in the 'By changing cell' box and select cell A2. In elementary algebra, the quadratic formula is a formula that provides the solution(s) to a quadratic equation. Python Program to Solve Quadratic Equation. Ex.: To find the roots of the equation x + 5x + 6 0, enter a 1, b 5 and c 6. calculator for solving quadratic equations allows you to find the roots of a quadratic equation with providing a detailed solution. Click in the 'To value' box and type 24.5Ĩ. To solve a 2 nd order equation like ax + bx + c 0, enter or replace the coefficients a, b and c. On the Data tab, in the Forecast group, click What-If Analysis.ħ. A quadratic equation can be solved in multiple ways, including factoring, using the quadratic formula, completing the square, or graphing. You can use Excel's Goal Seek feature to obtain the exact same result. But what if we want to know x for any given y? For example, y = 24.5.

[prosmains]

Peak width fityk

Peak width fityk software#

Peak width fityk plus#

With 82 nonlinear peak models to choose from, you’re almost guaranteed to find the best equation for your data. PeakFit gives the electrophoresis user the ability to quickly and easily separate, locate and measure up to 100 peaks (bands), even if they overlap. PeakFit can even deconvolve your spectral instrument response so that you can analyze your data without the smearing that your instrument introduces. Overall area is determined by integrating the peak equations in the entire model. As a product of the curve fitting process, PeakFit reports amplitude (intensity), area, center and width data for each peak.

Peak width fityk plus#

PeakFit includes 18 different nonlinear spectral application line shapes, including the Gaussian, the Lorentzian, and the Voigt, and even a Gaussian plus Compton Edge model for fitting Gamma Ray peaks. PeakFit lets you accurately detect, separate and quantify hidden peaks that standard instrumentation would miss. Suggested uses for PeakFit are represented below however, PeakFit can be used in any science, research or engineering discipline. PeakFit Is The Automatic Choice For Spectroscopy, Chromatography Or Electrophoresis Background Functions (10): Constant, Linear, Progressive Linear, Quadratic, Cubic, Logarithmic, Exponential, Power, Hyperbolic and Non-Parametric.Real-time Fitting in conjunction with data point selection, deselection.Automatic detection of baseline points by constant second derivatives.Extensive mathematical, statistical, Bessel, and logic functions.Estimates can contain formulas and constraints.Transition (14): Sigmoid Asc, Sigmoid Desc, GaussCum Asc, GaussCum Desc, LorentzCum Asc, LorentzCum Desc, LgstcDose Rsp Asc, LgstcDoseRsp Desc, LogNormCum Asc, LogNormCum Desc, ExtrValCum Asc, ExtrValCum Desc, PulseCum Asc, PulseCum Desc.General Peak (12): Erfc Pk, Pulse Pk, LDR Pk, Asym Lgstc Pk, Lgstc pow Pk, Pulse pow Pk, Pulse Wid2 Pk, Intermediate Pk, Sym Dbl Sigmoid, Sym Dbl GaussCum, Asym Dbl Sigmoid, Asym Dbl GaussCum.Statistical (31): Log Normal Amp, Log Normal Area, Logistic Amp, Logistic Area, Laplace Amp, Laplace Area, Extr Value Amp, Extr Value Area, Log Normal-4 Amp, Log Normal-4 Area, Eval4 Amp Tailed, Eval4 Area Tailed, Eval4 Amp Frtd, Eval4 Area Frtd, Gamma Amp, Gamma Area, Inv Gamma Amp, Inv Gamma Area, Weibull Amp, Weibull Area, Error Amp, Error Area, Chi-Sq Amp, Chi-Sq Area, Student t Amp, Student t Area, Beta Amp, Beta Area, F Variance Amp, F Variance Area, Pearson IV.Chromatography (8): HVL, NLC, Giddings, EMG, GMG, EMG+GMG, GEMG, GEMG 5-parm.Spectroscopy (18): Gauss Amp, Gauss Area, Lorentz Amp, Lorentz Area, Voigt Amp, Voigt Area, Voigt Amp Approx, Voigt Amp G/L, Voigt Area G/l, Gauss Cnstr Amp, Gauss Cnstr Area, Pearson VII Amp, Pearson VII Area, Gauss+Lor Area, Gauss*Lor, Gamma Ray, Compton Edge.

Peak width fityk software#

The Fitting Functions and Features for PeakFit by Systat Software are shown below: Only PeakFit offers so many different methods of data manipulation. And, PeakFit even has a digital data enhancer, which helps to analyze your sparse data. AI Experts throughout the smoothing options and other parts of the program automatically help you to set many adjustments. PeakFit also includes an automated FFT method as well as Gaussian convolution, the Savitzky-Golay method and the Loess algorithm for smoothing. This smoothing technique allows for superb noise reduction while maintaining the integrity of the original data stream. With PeakFit’s visual FFT filter, you can inspect your data stream in the Fourier domain and zero higher frequency points - and see your results immediately in the time-domain. PeakFit Offers Sophisticated Data Manipulation. PeakFit’s graphical placement options handle even the most complex peaks as smoothly as Gaussians. Each placed function has “anchors” that adjust even the most highly complex functions, automatically changing that function’s specific numeric parameters. If PeakFit’s auto-placement features fail on extremely complicated or noisy data, you can place and fit peaks graphically with only a few mouse clicks.

[lessons4-all]

Learn how to solve even the toughest math problems in 4 easy steps

In the subject of mathematics, there are numerous ways to approach a problem and come up with a solution. Even the trickiest math problem may be solved with this streamlined step-by-step method.

There are many ways to solve math problems, but a 4 -step method is a simplified method that may help anyone in tackling even the most difficult question.

The process is:

1. Visualize the problem 2. Approach to be followed for that problem 3. Lastly, solve the problem

Here are four steps to help solve any math problems easily:

1.     Carefully read, understand, and define the type of the problem

Check the nature of the problem when you first begin studying math to see if it is a word problem, a problem involving fractions, a problem involving quadratic equations, or any other form.Before continuing, specify the category that your mathematical issue falls in because doing so will help you come up with the best answer. It is crucial to read the problem carefully and make sure you have grasped it before moving on to the next stage.

2.     Draw and review your problem

Drawing the problem after you have comprehended it can be the following step because it will aid in your future planning. Simple shape drawings or shapes with numbers might be used for the drawing. You might also utilise graphs or look for patterns in this situation. After completing this comprehending, reading, and drawing process, you must review the analysis you came to. You can use this to decide the problem's kind and approach to solving it.

3.     Develop the plan to solve it

Here are four easy steps that must be followed in order to create a plan to fix it. The procedures are as follows:

·       The formula needed to solve the problem must first be determined. Reviewing the concepts from your textbooks will help you resolve this issue, so take your time.

·       To receive the solution to your issue, you must put your need in writing. To accomplish this, you must create a step-by-step list of the materials you will need to address the issue and maintain organisation.

·       If there is a simpler problem accessible, you should usually try to tackle that one first. Sometimes the formulas used to solve both problems are redundant. You'll have more time because of this to solve the challenging puzzle.

·       You can try to estimate the solution before beginning to solve it by making an educated guess about the solution. Here, you may see the quantity and other elements that will influence the same. Review the estimate once again to make sure you didn't forget anything.

4.     Solve the problem

You might begin tackling the problem after your plan and method are prepared. Following are the steps:

·       Make sure you follow all the instructions you listed to solve the problem. Verify the accuracy of each of your responses by crossing them out.

·       After you've finished every step, compare the result to the estimates you've stated. If the outcome is not what you were hoping for, you will have saved time. Additionally, make sure you thoroughly followed each step by checking.

·       You may always go back to the planning step and create a new plan if you find out in the middle of the process that your current one isn't working. This can occur occasionally as a result of typical blunders, but you should learn to accept it and be prepared with a Plan B to address it.

·       Once you've correctly addressed the issue, you should go back and review your approach. Think on the issue and the approach you used to resolve it for a moment. This will make it easier to determine the concepts you should study while exercising.

Are you looking for the best math tuition services in Singapore? You've come to the right place. Using Lessons4All, you can find the best math tuition center or tutor in Singapore.  Check out our website for more information