proof-by-intimidation

[proof-by-intimidation]

whenever a linear algebra professor catches you having too much fun with math they are legally obligated to make you do gram-schmidt as a way of humbling yourself

[proof-by-intimidation]

headache all day

drink water, eat food

hours pass

headache

watch category theory playlist by richard e borcherds

no more headache

[proof-by-intimidation]

Some mathematicians claim they can visualize R^4, or even higher-dimensional spaces. I think this is probably possible for the human mind to do, in principle; the human mind often proves surprisingly flexible. But I think it probably takes a lot of concerted practice, and because you are stretching your mind beyond its intended limits in a purely-internal, imaginative way with no external reference points to check against, I think it probably has some things in common with certain types of meditative practice.

It's also common to hear mathematicians say that if humans had visual intuition for dimensions higher than 3, higher-dimensional geometry may have advanced much farther, since visual intuition is so often crucial in thinking of proofs.

So, ok, I suspect that AI will obviate the utility of this before it could ever have the chance to get off the ground, but this all makes me imagine a world where techniques for learning to visualize higher dimensions are well-known and practiced, and have become a functional necessity for being a working geometer in higher-than-3-dimensions. And these techniques require a lot of persistent practice and training, which (by the nature of the thing) is hard to precisely communicate to students. So part of becoming a geometer involves training in what is basically a meditative practice, where, à la Zen, much of the process involves not directly teaching the student but giving them prompts and mental exercises that are meant to trigger internal, incommunicable revelation. But instead of enlightenment it's geometric intuition. And so if we're getting a math PhD in, say, differential equations or something, it's mostly like it is today. But if your PhD is in low-dimensional topology there's like a whole monastic apprenticeship style thing that just comes along with it. People sometimes drive themselves insane. People sometimes drive themselves insane.

[proof-by-intimidation]

I am wondering if anyone here can offer me a bit of insight into what the "big theorems" of algebraic geometry and/or algebraic topology obtained by infinity-categories are. The Beck's Monadicity Theorem's of the subject, so to speak. Just trying to get a feel of what the formalisms are building towards.

[proof-by-intimidation]

I've been thinking about A-infinity algebras and the homotopy transfer theorem a lot. There's this idea I've been working on and I now understand enough to be confident that my idea should work, but there are enough holes in my understanding that I can't quite write a proof. This is the part of math that is the most maddening. I want to just keep working until I've solved it but it'll probably still take dozens of hours until I have a solution. It's also the most physically exhausting part of the process. I can feel the gears in my head turning and it's so effortful

[proof-by-intimidation]

When you graduate from being interested in feet.

Is this anything?

[proof-by-intimidation]

As I resume my quest to become an algebraic geometer, I am awe struck by the sheer abundance of excellent resources available in terms of online lecture notes. I resumed my quest by purchasing Hartshorne, expecting to resume the struggle, but it turns out that is a very 2016-coded way of studying algebraic geometry.

What a wonderful world we are living in to have access to such resources so easily!

In particular I am really falling in love with the lecture notes of Gathmann.

[proof-by-intimidation]

My talk was uploaded on YouTube and one person commented (months later) that they found it useful. Ahhh, that was the whole point!! For a non-trivial number of people to get something out of it!

[proof-by-intimidation]

[proof-by-intimidation]

can't wait for vacation to resume the classic activity of "walk in some direction (not actually necessarily in one direction, can make any turns) aimlessly for hours until i am too tired, then rest and try to find my way back with no usage of phone maps"

[proof-by-intimidation]

My faith is based on only one axiom...... that every category is a locally small category in some Grothendieck universe, and therefore the Yoneda Lemma always applies......

[proof-by-intimidation]

Your personal rant is much appreciated. Thank you for the words in the end, they truly are comforting.

The PhD application process is so dreadful. And you are telling me I might have to do this again in 3 years for postdoc and again and again and again...

Part of me wants to quit this shit show. I love the idea of doing a phd but the application process makes me want to puke. What do you mean that I will have to vouch for myself, tell great things about me when I don't even believe it.

Moreover, getting a rejection hurts. Even when I know that there could be a lot of reasons for it, I can't help taking it personally.

Even when I try to distract myself by doing some math, I feel guilty that I am not doing enough for my applications. Maybe I should search more for open positions. Maybe I should change my cover letter completely. Maybe I should start cold emailing people.

Ugh, it's so frustrating.

[proof-by-intimidation]

The PhD application process is so dreadful. And you are telling me I might have to do this again in 3 years for postdoc and again and again and again...

Part of me wants to quit this shit show. I love the idea of doing a phd but the application process makes me want to puke. What do you mean that I will have to vouch for myself, tell great things about me when I don't even believe it.

Moreover, getting a rejection hurts. Even when I know that there could be a lot of reasons for it, I can't help taking it personally.

Even when I try to distract myself by doing some math, I feel guilty that I am not doing enough for my applications. Maybe I should search more for open positions. Maybe I should change my cover letter completely. Maybe I should start cold emailing people.

Ugh, it's so frustrating.

[proof-by-intimidation]

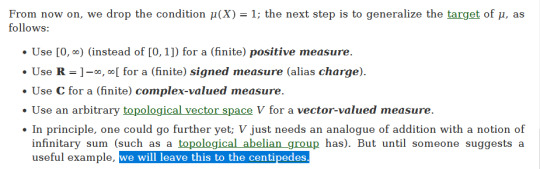
everytime i open ncatlab its a whole new experience

[proof-by-intimidation]

Failing my set theory seminar because I only studied videos from a beetlegirl on youtube

[proof-by-intimidation]

two words that really shouldn't be the same word:

inductive in "inductive reasoning" (philosophy term)

inductive in "inductive proof" (math term)

[proof-by-intimidation]

What is the proper pace for mathematical research, and how does one know if you are pushing too much versus relaxing too much? I feel that I have this narrative that things take time in my head, but I'm not sure how much that is becoming a self-fulfilling prophecy versus simple realism.