#testing polynomials

[artemistorm]

NoOoOoOo! That math final was awful 😭. I didn't finish and neither did 3/4 of the class. All the problems were super hard and it turns out I forgot how to factor polynomials. 😭😭

[moonknightproductions]

just did a bit of math for the first time in literal years and idk why I was putting it off so long polynomials and fractions are my best friends

[lavrach]

The worst feeling is finding out that the test, that you failed, would be easy if you studied for FIVE more minutes, specifically if you spent the five minutes studying the topic that "probably wasn't going to be on the test"

[strwbrythoughts]

time's up | dr. veritas ratio

The doctor - also known as your husband - has come to refine your problem-solving skills, particularly in mathematics.

A/N: This man is insufferable but I simp because smart + muscles = hot!!! Also can you see me projecting onto this and wdym i don't like triangles, ngl i accidentally switched to first person pov at the ending 💀 this is why i need to find time to write in my busy busy schedule

Divider by @/osqrie

"You have 15 minutes to solve these questions. You may begin."

The soft click of the digital stopwatch in his right hand sounded louder than ever to your ears. Your eyes went from his soft, smiling face - which in itself, was a rarity - towards the questions printed on the single sheet of white, A4-sized paper on your desk. They were all mathematics equations. Every single one was an integration-related question.

You always thought that you could escape the topics you didn't like after finishing your studies in the undergraduate level. Life had been pretty smooth-sailing after graduation; you opened a cute and cozy bakery, you married a genius, lived in a comfortable home, have and maintain a loving relationship...but this. This was the least of what you would expect. Being tutored and tested on the one subject you refused to take back in university.

Or perhaps it was your mistake when you told him your weak points in the academic aspect of yourself. That was a side effect of marrying a genius, you supposed...or a 'Mundanite' as he would use to refer to himself.

But you couldn't think of that now. You had a test to complete, and you didn't want to receive his punishment for not being able to do so within the given timeframe. His eyes could be felt on you, as if burning into your body and directly gazing at the very essence of your soul. The rise in your heartbeat and downturned gaze highlighted how nervous you felt to answer this paper.

After taking a deep breath to clear your head, you observed the questions carefully. There were 3 questions, and all of them were pretty easy to solve (or at least, that was what the instructions said). The first two questions were fine; only including polynomials and exponents respectively. As you got to the third question though...you couldn't say it was 'fine'.

It included one of your most disliked topics...trigonometry.

Granted, the trigonometry was pretty simple, but you never seemed to be able to wrap your head around how so many formulas could be derived from them. When Veritas explained it to you in one of your tutoring sessions, you asked for more breaks than usual since you had put a barrier in your mind; 'I will never understand this'; which in turn, made the topic seemed harder than it actually was.

The soft 'ding!' of the bell on my desk reminded you that you had five minutes left for this last question. You gulped, hoping your nervousness would be swallowed away as well. His eyes were glued to the back of your head; not that you dared to look behind my shoulder at the moment.

Every second counts after all, even if you had no clue what steps you should take to solve the problem on the paper.

Your brain seemed to malfunction and your memorisation skills failed you right this moment. You didn't remember the basic formulas, and you didn't know how to derive them from the triangles either. With no viable options left, you decided to unleash your expertise; cooking up your own theories.

'Ah, he's definitely punishing me for this...' was the only thought floating in your brain. Autopilot mode was switched on, and your hand glided across the paper, writing down whatever nonsense that seemed to be related to trigonometry, regardless whether they were correct or not.

The digital stopwatch in Veritas' hand had reached its last minute; the fifteenth minute. "Time's up," his voice bounced off the white walls right into your ears. "Put down your pen and stop writing."

Although reluctant, you did not wish for a heavier punishment. His words were followed by the sound of the pen being put onto the wooden desk. Within seconds, he was stood right next to your desk, using his index finger and thumb to pick up the piece of paper.

His eyes scanned my answers, going from left to right as he inspected each line of working. There was a faint smile on his lips...until it was gone.

Gulp.

He had definitely seen the absolute mess you made on the last question.

A slam onto the wooden desk; you swore you heard the wood crack a little bit. "Did I not teach you this last question?" You could barely reply. His tone was dripping with condescension, but you didn't take offence from it. Both you and him knew he had the right intentions, but his ways wouldn't be able to satisfy everyone.

"You did! I...I just didn't like it."

Veritas let out a 'tsk' thrice, seeming almost animated as he did so. "You do know what you have to do now, right? So come on, what are you waiting for? Is time not ticking?" He took a seat on his chair, eyes looking straight into mine.

You sighed, preparing your facial muscles...as you climbed into his lap. Ah yes, the 'one hundred kisses or you're not leaving this room' punishment. A classic, really. Your lips peppered his face, landing on each part like the first snow of winter; gentle and heartwarming.

"Lunchtime is nearing, so you better carry out your responsibility quickly and dutifully, dear. You'd hate to have lunch at 4 PM again, hm?"

Ah, crap! He was right! Better get to work now!

Thank you for reading!

[yoneda-emma]

is there a reasonably efficient way to check whether an arbitrary polynomial is convex? apparently deciding it for polynomials with degree higher than four in more than four variables is NP-Hard but I can't find anything conclusive for polynomials in a lower number of variables

obviously I could just sample a bunch of test points and get a probabilistic solution that way but that's so lame =w=

[fexiana]

EuphoriaSims: Fair Weather Friends

Summary: Rue invites Lexi to join her and Jules for a nice sunny day at the community pool, but their friendships are tested when Rue finds out Lexi and Fez are more than just dating.

Music Credits: Subterranean Homesick Alien (instrumental version/normal version) by Radiohead and Polynomial C by Aphex Twin

Thank you to animation creators VMS, Purpura Sims, Blue Simka, Valeria Nice, Void Archer, @mishtern, Ideal SimsFour, @Stevenstudios, WW animators, and @alexisarielgaming for the city pool build as I wouldn't have been able to portray my story without them.

If you like this episode, please like, reblog, or leave a comment. Thanks for watching 🧡

[merpmonde]

Sangaku Saturday #13

Last week, we uncovered this configuration which is also a solution to our "three circles in a triangle" problem, just not the one we were hoping for.

This is something that happens in all isosceles triangles. Draw the inscribed circle, with centre B, and the circle with centre C, tangent to the extended base (ON) and the side [SN] at the same point as the first circle is. Then it can be proved that the circle with centre A, whose diameter completes the height [SO] as our problem demands, is tangent to the circle with centre C.

But that's not what I'm going to concentrate on. Despite this plot twist, we are actually very close to getting what we want. What the above configuration means is that, returning to the initial scaled situation with SO = h = 1 and ON = b, we get

Knowing a solution to a degree 3 equation is extremely powerful, as we can factor the polynomial and leave a degree 2 equation, which has simple formulas for solutions. So, to finish off, can you:

1: prove that

2: solve the equation 2x²-(s-b)x-1 = 0, and deduce the general formulas for p, q and r that fit the configuration we are aiming for;

3: test the formulas for an equilateral triangle, in which s = 2b.

This last question is the one the sangaku tablet claims to solve.

[uncertain-noob]

Continued Fractions of Polynomial Roots

Imagine you've got a polynomial, and you want the continued fraction representation of one of its roots. First things first, if you count the sign changes in the coefficients as you go from highest to lowest, you've got an upper bound on the number of roots that poly has that are positive. If the number of changes is 1 or 0, the bound is exact. This is due to Descartes. Much more here https://en.wikipedia.org/wiki/Geometrical_properties_of_polynomial_roots Now, imagine the root you care about is the largest positive root. We want to find its integer part to start our continued fraction journey. If we shift the poly by mapping x to x+1, we move that root one step towards the negative ie we subtract one from the value of its highest root. If we keep doing this, Descartes test tells us that eventually all the coefficients of the poly will have one sign, and the root will have changed from positive to negative. If we backtrack one step, we know the largest integer shift which still has one positive root. The root of that shifted poly will be the fractional part of the root we cared about, and the amount we shifted by is the first number in our continued fraction. Okay, but that's only the first term. Yeah, but all you have to do now is take your poly, substitute x -> 1/x and then multiply by x^n, where n is the degree, to get back a poly in x, whose roots are all the inverses of the roots before we transformed. And the root we care about, the inverse of the fractional part of the root we first cared about, is the only, and largest, root of the poly greater than 1. The other roots, after a few iterations, all end up in the unit disc with negative real part. This is stuff i read here https://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.135.107&rep=rep1&type=pdf but i think its pretty well a 'folk theorem' ie, so many dudes worked it out more or less at the same time, and it just sort of moved into the general conscience. But I could be wrong. Also, for anyone implementing with a machine, some caveats. The coefficients of the poly are not well controlled. To get more than a few dozen partial quotients in the cf, you'll need arbitrary precision integers, like scala's BigInt. I shifted by increasing powers of 2, tested how many roots i had, and then used a binary search after i had an upper bound. Mostly partial quotients are low, so there just stepping through might be smarter. See the Gauss Kuzmin distribution for further guidance. https://mathworld.wolfram.com/Gauss-KuzminDistribution.html

[spine-buster]

So High School ft. Adam Cole | Chapter 4

The days and nights passed, as they always did.  Kyle and Janie’s visit brought some much needed positivity in the household, despite the days leading up to Austin’s surgery.  He wouldn’t admit it out loud, but he was nervous.  Maybe not as nervous as when he had his concussion and was checking in with the brain doctor three times a week, but nervous nonetheless.  The surgery wouldn’t take more than an hour.  He could start physiotherapy three weeks later, which was a blessing because he was becoming a bit stir crazy not being able to go to the gym.  But anytime he had to be put under, he got nervous. 

“D’you want to watch anything before bed?” Violet asked as she emerged from their ensuite, switching off the light.

“No, I’m good,” Austin shook his head.  “I want to get a good night’s sleep before the surgery.  Well, at least try to.”

As Violet got into bed, she got as close as she could to him before giving him a few quick, light kisses.  “I’ll make sure I’m the last person you see before you go under and the first person you see when you wake up.”

Austin smiled.  “I’m gonna wake up high thinking you did the surgery and that’ll scare me shitless.”

Violet snorted and they both began giggling, lightening up the mood.  “God knows med school wasn’t my calling,” she quipped.

“I think you did pretty good regardless.”

“I have you, don’t I?”

Austin smiled.  He loved her so fucking much.  “C’mere,” he whispered, wanting her close again so he could kiss her one, two, three times, each time longer than the last.  “I love you.”

Violet kissed him again, and again, and again – hands wandering, tongues making a mess.  It wasn’t long before her hand made it under the waistband of Austin’s boxers.  “Let me show you how much I love you.” 

***

On Tuesday after school, as Violet was making her way to the bus stop, she saw Austin waiting too, but noticed his demeanour was completely different than how she knew it.  He was angry.  Not just angry – pissed.   She made it just in time to get on the bus, and they took their usual seats, Austin letting her get into the window seat before sitting beside her.  “Is everything okay?” she asked, worried.

“No, everything is not okay,” he grumbled.

“What’s wrong?”

He sighed.  “Stupid math.”

“What happened?”

“I failed my last test on polynomial graphs,” he revealed.  “My teacher says if I don’t get at least a 70 on the next test my mark is gonna dip below a C, and I can’t – my parents have this stipulation that all my grades have to be above a C or else they don’t let me go to wrestling shows in Philly.”

After their conversation at Francesco’s after the dreadful party, she knew and understood how important that was to Austin.  “I can help,” she offered.

Austin was still in his own world, though.  Still spiralling a bit.  “Not to mention that if my overall average isn’t high enough, Manheim Community Centre won’t hire me in the spring and summer to do swimming lessons as part of their student hiring program, and I really need that job—”

“—Austin.”

He snapped out of it.  “What?”

“I can help you,” Violet repeated.  “When’s your test?”

“Friday.”

“That means we have three days to study,” she said assertively.  “We’ll study at your house every day after school until the test, until you’re confident you can get that mark.”

Austin was flabbergasted.  None of his other friends had volunteered their time or energy to help him.  Violet was the only one who was going to willingly take time out of her own schedule and homework – which was more academically challenging than his, no doubt – to help him, all so he could get a C.  “You’d do that for me?”

“We’re friends, aren’t we?” she quipped back.  “Besides, I know now how much wrestling means to you.  You shouldn’t not go see shows just because of a math mark.  I mean, I understand why your parents have that stipulation – mine do too – but math shouldn’t get in the way of it.”

Austin nodded.  There was a moment of silence between them as he realized the magnitude of Violet’s generosity.  “What’s your parents’ stipulation?” he asked.

“Straight As are a non-negotiable.  That and at least two extra-curriculars.  That’s why I’m so involved in student government and the school newspaper,” she revealed.  “Plus, I do this language program at home – you know how like, kids will go to Greek school or Hebrew school on the weekends?  Well mine is online and it’s basically German school.  That way I can speak to my grandparents.  Oh, and I play tennis.”

Austin felt his head spin at the thought of everything she had to do on top of all her regular school work.  Jugging all that with expectations of getting into an Ivy League like UPenn or a university like Georgetown?  He’d be shitting himself.  “Does it ever get hard for you…you know, balancing everything?”

Violet bit her bottom lip and averted her eyes.  “I mean, yeah, sometimes,” she mumbled out, as if she didn’t want to.  She knew she had to be honest with him because he was only ever honest with her.  “It can be a lot, like when things start to add up over the week, especially when I want to have a social life, you know?  Like at the end of the day I’m just a teenage girl.  I want to be out with my friends and at the same parties they are.”

Austin nodded.  “Yeah, I get it.”

“I think it’s harder because I’m an only child,” she continued.  He noticed that she was picking at her signature red nail polish.  “They just have high hopes for me.  I get it, I really do.  I just want a bit of a break sometimes.  The world won’t end if I get a B on a French vocabulary test, you know?”

Austin gave her a smile.  “I know,” he nodded.  “The world won’t end if I get below a C in math, either.”

He raised his hand and pushed hers apart playfully, making sure she stopped picking at her nails.  She looked at him finally, giving him a smile, stopping her nervous habit.  Her hands were as soft as Austin remembered them.

When they arrived at his usual bus stop, they got off together and he led the way to his house, which was only a short walk down the road.  As they approached the small yellow bungalow, Austin got nervous.  Having seen even just the outside and front foyer of Violet’s house, the difference was night at day.  He was pretty sure three of his houses could fit in her house.  When he looked over at her, she was smiling.  “Your house is beautiful,” she said, with such genuine earnestness that he knew she meant it.  “I love the yellow.”

“My grandma’s gonna be home,” he didn’t know what else to say.  He didn’t know why he was so nervous.

“That’s cool.  I’m a grandma whisperer too,” she winked.

When they walked in, Violet could hear the sound of a TV being on.  She slipped off her Uggs and followed Austin around the entrance wall that was covered in family photos.  Photos from family vacations to the beach, Washington D.C., and some other places Violet couldn’t quite make out.  “Nana, I’m home,” Austin called out.

Violet saw his grandma on the couch watching TV, and once she noticed a girl in a very formal Catholic school uniform was with her grandson, a highly bemused look adorned her face.  “Hi sweetie,” his grandma greeted.  “How was school today?”

“It was alright.  Same old, same old.”

“And who is this?”

Austin knew he wouldn’t have to do any introductions.  If the late night phone call with his mom was any indication, Violet could hold her own.  He didn’t know many people who were as self-assured as she was.  “Hello, it’s very nice to meet you,” Violet began, approaching the couch with her hand extended so grandma wouldn’t have to get up.  “I’m Violet Schwarzkopf, one of Austin’s friends.”

“Nice to meet you.  I’m Austin’s grandmother Judy,” she said.  “That uniform doesn’t look like you go to Lancaster West.”

“Oh, no ma’am,” Violet shook her head, smiling a little.  She broke out the ma’am – Austin had to hold back his own laugher.  “I actually go to St. Anne’s Academy, down the street from Lancaster West.  I’m just here to help Austin with some Algebra 2 homework for his test on Friday.  I promise we’ll be out of your way and won’t be too loud.”

Nana smiled.  Austin smiled at her smiling.  Clearly Violet was making a great first impression.  “That’s quite alright, dear.  Anything to help Austin get the grades he needs to do the things he wants,” she gave her grandson a knowing look.  “You’ll be fine to go in the kitchen then…the TV won’t be too loud in there.”

“Do you need anything while you’re in there?  A glass of water?  Some snacks?” Violet asked.

“Do you know how to make a gin martini?” Judy quipped.  Everybody laughed.  “I’ll be fine.  You two go ahead and study.”

Austin led Violet through to the kitchen, a small U-shaped space with old wood cabinets and a big window overlooking part of the yard.  There was a round table against the wall with no cabinets.  Violet sat at the table while Austin went into the fridge.  “Want anything?” he asked.

“Where have you guys gone on family trips?” she completely ignored his question, looking instead at some more of the family photos on the wall above the table. 

Austin glanced over from behind the fridge door.  “Um, before my parents divorced, we used to drive down to Myrtle Beach every summer and spend a couple of weeks there,” he said.  “My dad took my brother and I to D.C. a few summers ago, and my mom took us to New York the summer after that.”

“That’s so cool,” Violet smiled.  “D.C.’s pretty awesome, isn’t it?  I love the Georgetown neighbourhood.”

“What about your family?”

“We’re pretty big skiers, so a lot of our trips revolve around skiing.  Usually during Christmas Break we head somewhere like Whistler or Mont Tremblant or Telluride.  Then in the summers its always about two weeks in Toronto so my mom can see her family, then we fly to Berlin so my dad can see his family.  After that we spend time in different places like Italy or Spain or Portugal.”

The second the words finished coming out of her mouth, Violet regretted ever saying them out loud.  These were rich people things that rich people did.  Not everybody skied.  Not everybody summered in Europe.  Not everybody had the advantages that her family had, and she needed to learn once and for all that not everybody had the same life experiences she had.  To say these things out loud like they were nothing, with such nonchalance as if everybody did them – who did she think she was?

“That sounds really cool,” Austin finally said, closing the fridge.

“Sorry.”

“Why are you apologizing?”

“I know that just made me sound like a stuck up brat.”

“No it didn’t,” he assured her, handing her a bottle of blue Gatorade.  “I asked you a question and you answered it.”

“Yeah, but—”

“—But nothing,” he interrupted.  “I know your family is richer than mine, Violet.  That’s not a secret.”

Her stomach churned.  “I just don’t want it to be something that comes between us,” she admitted out loud, in a voice that was low and vulnerable. 

“Vi…” Austin cooed, pulling up a chair to sit, getting as close to her as he could without it being inappropriate.  “If it was something that could, it would have already.  It won’t.  It’s not going to.  I promise.  Do you?”

She was barely breathing from being so close to him.  What was this?  What was she feeling?  “I promise,” she nodded.

“Good,” he smiled at her, and she could see his dimples and the crinkle of his eyes.  “Now can you help me with Algebra 2?”

They took out the textbooks and got down to business, going over concepts and equations.  She looked over his test to see exactly where he went wrong.  Violet even made up equations for him to practice on the spot, which freaked him out.  Slowly but surely, though, he was getting it.  Violet was definitely a much better and clearer explainer than his teacher at Lancaster West.  She made sure he followed things step by step, and would check each time to make sure he was doing it right.  It helped that she smelled good and was so pretty.

Wait, what?

Before he knew it, he heard his mom walking through the door.  His younger brother was louder than she was, stomping through the house loudly and saying hi to Judy before his footsteps could be heard walking towards the kitchen.  When he saw Austin sitting at the table with Violet, he stopped dead in his tracks.  He looked like a deer caught in the headlights.  “Whoa.”

His brother still thought girls had cooties.  Austin rolled his eyes at his reaction.  “Hello to you too, Brent.”

“Austin?” he heard his mom call.

“I’m in the kitchen studying!” he called back, knowing that would get a reaction out of her.

“Studying?” his mother was clearly confused.  A few moments later, she appeared right behind Brent.  She tried not to let the shock and confusion overcome her face, but it was no use.  Her son was studying?  And there was a girl over?  “Oh, hello!” she greeted warmly as she saw Violet.

Violet immediately got up from her seat.  “Hello Ms. Jenkins.  It’s nice to finally meet you.  I’m Violet Schwarzkopf – we spoke on the phone a couple of weeks ago,” she extended her hand.

“I remember.  It’s nice to finally meet you as well.  Call me Cathy,” she shook her hand before looking over at her son.  “Special occasion?”

“Violet’s helping me with Algebra 2.  I have another test on Friday.”

“I hope that’s alright,” Violet interjected.

“Anything to get Austin studying,” Cathy quipped.  “Are you staying for dinner, Violet?”

“Oh no no no, no thank you.  I wouldn’t want to intrude like that.  Plus, on the days my dad has to stay in Philadelphia to lecture, my mom gets lonely and would want me home for dinner.”

Cathy made a mental note to ask about that sometime later.  Maybe she’d ask Austin.  “Well, the car is still warm, so I will at least drive you.  Where do you live?”

“In The Berries.”

Cranberry Street, Blueberry Street, Blackberry Street, and Mulberry Street – Cathy knew the area well.  Who would have thought her son would have a friend who lived in The Berries?  Violet got ready quickly – Austin too, since he was adamant on coming – and they drive through the streets of Manheim before reaching her house.  Violet made polite conversation and mentioned she’d be back the rest of the week to tutor to help Austin pass his test.  Cathy was impressed with the size of the house when she pulled into the driveway, waiting until Violet was inside before backing out.

As she put the car in drive, she looked at her son.  “What?” he asked.

“Oh, Austin.”

___

The following Monday, Violet couldn’t get to the bus stop after school quickly enough.  Austin told her Friday that he thought the test had gone well, and she was eager to see if he got his mark back.  She ran as fast as her Uggs would take her, now carrying a heavier load with the introduction of her winter jacket, too.  When she finally arrived, she was almost out of breath.  “Did you get it back yet?” she huffed.

Austin had the greatest poker face known to man.  She didn’t even notice that he had something in his hand to show her.  He flashed whatever he was holding in front of her.  Violet say a big red ‘75%’ on the top right corner.

She screamed.  Subconsciously, she did what she did with all her friends whenever they aced something: she extended her arms up in jubilation, then out, pulling him in for a hug.  A giant smile took over his face as he reciprocated, bringing her close and squeezing her so hard he lifted her up off the sidewalk.  “I couldn’t have done it without you,” he said once he set her down.  “I’m totally serious.  I’d be failing without you.”

“I didn’t write that test, you did!” she spun it back to him.

“My parents are gonna be so happy, you have no idea.”

“I bet.  Is there a wrestling show coming up that this saves?”

He looked at her.  He couldn’t believe she was hanging on to that the way she was.  It meant the world to him.  “Yeah.  Yeah, absolutely,” he stuttered out.  “Listen.  I have a US History test coming up.  I could really use your help again.  You just explain everything so easy.  Can you come over Friday night so we can have a big study session?  I can still bring you home by curfew.”

Friday night.  Her parents would be away at a Christmas dinner for the UPenn faculty in Philadelphia for the night, so she’d be home alone Friday night and much of Saturday since they’d be spending the day in the city.  She would have to ask their permission, but she didn’t see why they would say no, especially if it was tutoring.  “I’ll ask my parents, but I should be good.”

Austin smiled from ear to ear, his breath catching in his throat.  “Great.”

[hiimtracher]

i need to become a math nerd to live on and it's just so hawwrdd

god damn i am already a nerd in every school thing exept math and geographics

even chemistry is getting back where it was in primary if not a bit worse

like it's hard because i mess up - es and + es in functions and i am currently going to have a test about polynomials and i try to study but i just can't get it right and for gods sake i know all the calculations but i still make major mistakes

HELP ME I NEED TO SEE THEM BUT I DON'T

[underoospeterparker]

I HATE being sick and having a test in five days because my brain was not working at all this entire week and now I have to cram revision for the entire functions unit except polynomials & permutations and combinations

[shummthechumm]

wish me luck people i have a test on factoring polynomials and am only 70% sure of what im doing!!!

[mariacallous]

In our increasingly digital lives, security depends on cryptography. Send a private message or pay a bill online, and you’re relying on algorithms designed to keep your data secret. Naturally, some people want to uncover those secrets—so researchers work to test the strength of these systems to make sure they won’t crumble at the hands of a clever attacker.

One important tool in this work is the LLL algorithm, named after the researchers who published it in 1982—Arjen Lenstra, Hendrik Lenstra Jr. and László Lovász. LLL, along with its many descendants, can break cryptographic schemes in some cases; studying how they behave helps researchers design systems that are less vulnerable to attack. And the algorithm’s talents stretch beyond cryptography: It’s also a useful tool in advanced mathematical arenas such as computational number theory.

Over the years, researchers have honed variants of LLL to make the approach more practical—but only up to a point. Now, a pair of cryptographers have built a new LLL-style algorithm with a significant boost in efficiency. The new technique, which won the Best Paper award at the 2023 International Cryptology Conference, widens the range of scenarios in which computer scientists and mathematicians can feasibly use LLL-like approaches.

“It was really exciting,” said Chris Peikert, a cryptographer at the University of Michigan who was not involved in the paper. The tool has been the focus of study for decades, he said. “It’s always nice when a target that has been worked on for so long … shows that there’s still surprises to be found.”

LLL-type algorithms operate in the world of lattices: infinite collections of regularly spaced points. As one way of visualizing this, imagine you’re tiling a floor. You could cover it in square tiles, and the corners of those tiles would make up one lattice. Alternatively, you could choose a different tile shape—say, a long parallelogram—to create a different lattice.

A lattice can be described using its “basis.” This is a set of vectors (essentially, lists of numbers) that you can combine in different ways to get every point in the lattice. Let’s imagine a lattice with a basis consisting of two vectors: [3, 2] and [1, 4]. The lattice is just all the points you can reach by adding and subtracting copies of those vectors.

That pair of vectors isn’t the lattice’s only basis. Every lattice with at least two dimensions has infinitely many possible bases. But not all bases are created equal. A basis whose vectors are shorter and closer to right angles with one another is usually easier to work with and more useful for solving some computational problems, so researchers call those bases “good.” An example of this is the pair of blue vectors in the figure below. Bases consisting of longer and less orthogonal vectors—like the red vectors—can be considered “bad.”

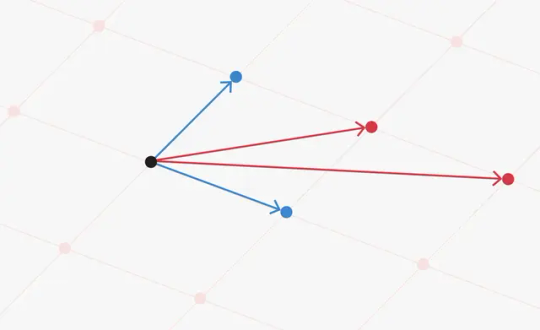
This is a job for LLL: Give it (or its brethren) a basis of a multidimensional lattice, and it’ll spit out a better one. This process is known as lattice basis reduction.

What does this all have to do with cryptography? It turns out that the task of breaking a cryptographic system can, in some cases, be recast as another problem: finding a relatively short vector in a lattice. And sometimes, that vector can be plucked from the reduced basis generated by an LLL-style algorithm. This strategy has helped researchers topple systems that, on the surface, appear to have little to do with lattices.

In a theoretical sense, the original LLL algorithm runs quickly: The time it takes to run doesn’t scale exponentially with the size of the input—that is, the dimension of the lattice and the size (in bits) of the numbers in the basis vectors. But it does increase as a polynomial function, and “if you actually want to do it, polynomial time is not always so feasible,” said Léo Ducas, a cryptographer at the national research institute CWI in the Netherlands.

In practice, this means that the original LLL algorithm can’t handle inputs that are too large. “Mathematicians and cryptographers wanted the ability to do more,” said Keegan Ryan, a doctoral student at the University of California, San Diego. Researchers worked to optimize LLL-style algorithms to accommodate bigger inputs, often achieving good performance. Still, some tasks have remained stubbornly out of reach.

The new paper, authored by Ryan and his adviser, Nadia Heninger, combines multiple strategies to improve the efficiency of its LLL-style algorithm. For one thing, the technique uses a recursive structure that breaks the task down into smaller chunks. For another, the algorithm carefully manages the precision of the numbers involved, finding a balance between speed and a correct result. The new work makes it feasible for researchers to reduce the bases of lattices with thousands of dimensions.

Past work has followed a similar approach: A 2021 paper also combines recursion and precision management to make quick work of large lattices, but it worked only for specific kinds of lattices, and not all the ones that are important in cryptography. The new algorithm behaves well on a much broader range. “I’m really happy someone did it,” said Thomas Espitau, a cryptography researcher at the company PQShield and an author of the 2021 version. His team’s work offered a “proof of concept,” he said; the new result shows that “you can do very fast lattice reduction in a sound way.”

The new technique has already started to prove useful. Aurel Page, a mathematician with the French national research institute Inria, said that he and his team have put an adaptation of the algorithm to work on some computational number theory tasks.

LLL-style algorithms can also play a role in research related to lattice-based cryptography systems designed to remain secure even in a future with powerful quantum computers. They don’t pose a threat to such systems, since taking them down requires finding shorter vectors than these algorithms can achieve. But the best attacks researchers know of use an LLL-style algorithm as a “basic building block,” said Wessel van Woerden, a cryptographer at the University of Bordeaux. In practical experiments to study these attacks, that building block can slow everything down. Using the new tool, researchers may be able to expand the range of experiments they can run on the attack algorithms, offering a clearer picture of how they perform.

[er-cryptid]

Testing if 1 and -1 are Zeroes

-- to see if x = 1 is a zero, sum up all the coefficients of the polynomial

-- if it equals 0, then x = 1 is a zero

Example: x^3 + 3x^2 - 25x + 21 +1 +3 -25 + 21 = 0 so 1 is a zero

-- to see if x = -1 is a zero, change the x to -x and sum up all the coefficients

-- if it equals 0, then x = -1 is a zero

Example: x^3 + 3x^2 - 25x + 21 -x^3 + 3x^2 + 25x + 21 -1 +3 +25 +21 = 48 so -1 is not a zero

.

Patreon

[machine-saint]

a thing I've seen is when people talk about how "continental" philosophy just seems like obfuscating words (this shows up a lot with Deleuze, Lacan, etc) people will compare it to how e.g. particle physics papers sound like total nonsense unless you're educated in the domain. and this is true, but i think the big difference is that physics is generally amenable to empirical testing. it doesn't matter if i understand your theory; if you can make predictions and I can verify them then I can be convinced that you're on to something. mathematics isn't empirical of course but you can sort of view, e.g., the quadratic formula as a thing that gives predictions about the roots of certain polynomials. (iirc back in the day when math culture was more secretive people would actually say 'i have a formula to solve cubic equations, i won't tell you but if you give me a cubic I'll solve it' or whatever)

but when I read Deleuze or similar things I'm just lost because there doesn't seem any way to verify this, it just seems difficult to reduce this into statements about the material world as opposed to just vibes.

analytical philosophy also can have issues with not being reducible to statements about the material world but at least i can understand what e.g. mereological nihilism means