#Interactive Math
Explore tagged Tumblr posts
Text
Systems of Equations: MEGA Teacher Resource with TONS of Engaging Activities
#systems of equations#algebra 1#algebra 2#solving systems#graphing#substitution#elimination#cramers rule#pixel art math#TI-84 calculator#math puzzles#desmos math#task cards#digital math activity#self-checking math#maze worksheet#interactive math#secondary math
2 notes
·
View notes
Text
In the vibrant community of early childhood education in Alpharetta, Georgia, fostering foundational math skills is crucial for young learners. At Science Akadémeia, the Atlanta Preschool of Science (SATAPOS), our summer programs focus on integrating math into daily activities to ensure children develop a strong understanding of numbers, patterns, and problem-solving techniques.
0 notes
Text


*its robin btw
#Clark trying to do the math in his head about how Bruce would even have a kid#Bruce does not acknowledge this at all after that interaction and Clark is afraid to ask#my art#superman#Batman#the Batman#Clark Kent#Bruce Wayne#robin#superbat
6K notes
·
View notes
Text

They’ve been on my mind lately
#digital art#my art#digital fanart#hermitcraft#hermitcraft fanart#mcyt fanart#hc designs#pearlescentmoon#pearlecentmoon fanart#ethoslab#etho fanart#tangotek#tango fanart#I have a maths test tomorrow but this is more important#I just wanna see more of their interactions
3K notes
·
View notes
Text
oh cool! 'girl math' is trending, I'm sure it's a celebration of the wonderful mathematical discoveries made by wom.. oh
oh wait
ok no nevermind
#radblr#radical feminism#radical feminists do interact#feminism#radical feminist safe#radical feminists please touch#women in stem#girl math
297 notes
·
View notes
Text








In which Ford struggles so badly to relate to other people that he wonders if he’s really human at all. The more isolated he becomes, the harder it is to reconcile with his own humanity.
#my art#gravity falls#Stanford pines#ford pines#bill cipher#comic#eye strain#TIME TO DUMP EVERY ONE OF THE 27483949 THOUGHTS IVE HAD INTO THE TAGS BABY#OK!! SO!!!!#I feel like Ford would wonder why he and Stan (being identical twins) aren’t. yk. identical. shouldn’t Stan have polydactyly too?#as a kid he would dream about secretly being nonhuman and being whisked away to a fantastical world full of people like him#finally free of new jersey‚ finally somewhere he belongs#a lot of this disconnect from humanity came from utterly failing at social interactions while others (including stan) navigated them easily#the feeling waned after Stan was kicked out and he didn't have that direct comparison but it never left#then out in the wilderness of gravity falls‚ his isolation and immersion in Weirdness dragged it back up to the forefront#he deserves to have a breakdown over questioning his own nature. as a treat <3#color symbolism time bc I have a problem and use it at every available moment!!! blue and yellow get more vivid#the further from humanity the subject is#bill is entirely made w pure rgb blue and yellow (+ approximately 2674835 textures/layers/blending modes. I reached 150+ layers. help)#I like the idea that he would appear to ford like pure math considering hes a geometrical motherfucker and how the rest of the mindscape wa#I tried to mostly use trigonometry and related stuff for the Math Greebling. as well as fractals i love you forever fractals#MORE SYMBOLISM:#the grid-ish diamond pattern in all of the mindscape bgs (and elsewhere) is a penrose diagram of spacetime#which shows other universes on the other sides of black holes#SOMEONE ASK ME ABOUT MY EUCLYDIA HEADCANON LATER. IVE DUMPED ENOUGH DUMB HCS IN THESE TAGS ALREADY#BUT I THINK ITS VERY FUN#anyways. fuckt up guys n their egos influencing how they view humanity. bill tells ford hes as human as they come bc he was so easily foole#ford cant reconcile with his humanity bc of a failure to perform in one area#and then the immense guilt and shame over what hes done <3#I have So many ford characterization thoughts. no man nor god can stop me
192 notes
·
View notes
Note
#C thinks MC is very attractive #that's for sure
Really? 👀 Was that as soon as they saw them or something they (reluctantly) realised over time? Or maybe when they saw them dressed up for an event, like prom or homecoming?
y’all, this grumpy baguette with a chihuahua’s rage absolutely hated MC’s existence at first and would’ve throttled them by their own two hands 😭 they had no time to stop and take in the face card or the mostly impeccable charm while they were getting demolished academically left, right, and centre.
the attraction started full-force (although you couldn’t waterboard this information out of C) when they started high school. by this point C had no interest in romance or relationships cause they ranked far below their actual priorities. i’d say the only person they found themself wanting to date, like ever, was MC 🤭
for romanced C, being MC’s homecoming date was the equivalent of dating your crush in high school. their feelings were all over the place but they couldn’t deny that they were much happier about it than they’d ever admit.
#when your first interaction with you soulmate is them imagining they’re strangling you in math class 😍#ro: c lacroix#if: the ballad of the young gods#interactive fiction#interactive novel#twine wip#interactive story
159 notes
·
View notes
Text

The Painted Lady turned around slowly, deliberately, and stared right through each ghost present in the room. Then, she raised her hands in a graceful arc, pointed loosely at the ceiling, and chanted, “Well, off you go.” She made a shoo, go away gesture, the spirits disappeared, and—wait. The spirits disappeared.
Izumi comes back in For the Spirits Chapter VI: Dream of You. Zuko has a mild panic attack and her upbeat attitude does not help him (or does it?).
Just what is she up to? And what is the meaning behind the blue eyes from Zuko's dream?
#zutara#atla#zuko#avatar the last airbender#atla fanart#prince zuko#zutara au#atla art#for the spirits#Chapter VI: Dream of You#the painted lady#painted lady#atla izumi#izumi#Izumi of Jang Hui#atla zuko#zuko art#zuko fanart#spirit touched zuko#new gods au#atla fanfic#atla fic#zuko fanfic#book one zuko#The Painted Lady's outfit design changes every time I draw her lol. Let's chalk it up to spirit shenanigans and leave it there.#I'm so excited about this chapter! It's most probably one of the most visual chapters I've written so far.#I couldn't decide between drawing this particular scene or two more... So I did all three *insert evil cackle*#Izumi is in a good mood for the entire chapter. Zuko has a panic attack for the entire chapter. Do the math.#This particular interaction is a Big Moment for him. You'll see why soon enough (*cough* next chapter *cough*)#For all the Lu Ten fans out there. I have an announcement! Our dearest boy Ten Ten is coming back in the next chapter. Keep your eyes open!
370 notes
·
View notes
Note
what's the 3-dimensional number thing?
Well I'm glad you asked! For those confused, this is referring to my claim that "my favorite multiplication equation is 3 × 5 = 15 because it's the reason you can't make a three-dimensional number system" from back in this post. Now, this is gonna be a bit of a journey, so buckle up.
Part One: Numbers in Space
First of all, what do I mean by a three-dimensional number system? We say that the complex numbers are two-dimensional, and that the quaternions are four-dimensional, but what do we mean by these things? There's a few potential answers to this question, but for our purposes we'll take the following narrative:
Complex numbers can be written in the form (a+bi), where a and b are real numbers. For the variable-averse, this just means we have things like (3+6i) and (5-2i) and (-8+3i). Some amount of "units" (that is, ones), and some amount of i's.
Most people are happy to stop here and say "well, there's two numbers that you're using, so that's two dimensions, ho hum". I think that's underselling it, though, since there's something nontrivial and super cool happening here. See, each complex number has an "absolute value", which is its distance from zero. If you imagine "3+6i" to mean "three meters East and six meters North", then the distance to that point will be 6.708 meters. We say the absolute value of (3+6i), which is written like |3+6i|, is equal to 6.708. Similarly, interpreting "5-2i" to mean "five meters East and two meters South" we get that |5-2i| = 5.385.
The neat thing about this is that absolute values multiply really nicely. For example, the two numbers above multiply to give (3+6i) × (5-2i) = (27+24i) which has a length of 36.124. What's impressive is that this length is the product of our original lengths: 36.124 = 6.708 × 5.385. (Okay technically this is not true due to rounding but for the full values it is true.)
This is what we're going to say is necessary to for a number system to accurately represent a space. You need the numbers to have lengths corresponding to actual lengths in space, and you need those lengths to be "multiplicative", which just means it does the thing we just saw. (That is, when you multiply two numbers, their lengths are multiplied as well.)
There's still of course the question of what "actual lengths in space" means, but we can just use the usual Euclidean method of measurement. So, |3+6i| = √(3²+6²) and |5-2i| = √(5²+2²). This extends directly to the quaternions, which are written as (a+bi+cj+dk) for real numbers a, b, c, d. (Don't worry about what j and k mean if you don't know; it turns out not to really matter here.) The length of the quaternion 4+3i-7j+4k can be calculated like |4+3i-7j+4k| = √(4²+3²+7²+4²) = 9.486 and similarly for other points in "four-dimensional space". These are the kinds of number systems we're looking for.
[To be explicit, for those who know the words: What we are looking for is a vector algebra over the real numbers with a prescribed basis under which the Euclidean norm is multiplicative and the integer lattice forms a subring.]
Part Two: Sums of Squares
Now for something completely different. Have you ever thought about which numbers are the sum of two perfect squares? Thirteen works, for example, since 13 = 3² + 2². So does thirty-two, since 32 = 4² + 4². The squares themselves also work, since zero exists: 49 = 7² + 0². But there are some numbers, like three and six, which can't be written as a sum of two squares no matter how hard you try. (It's pretty easy to check this yourself; there aren't too many possibilities.)
Are there any patterns to which numbers are a sum of two squares and which are not? Yeah, loads. We're going to look at a particularly interesting one: Let's say a number is "S2" if it's a sum of two squares. (This thing where you just kinda invent new terminology for your situation is common in math. "S2" should be thought of as an adjective, like "orange" or "alphabetical".) Then here's the neat thing: If two numbers are S2 then their product is S2 as well.
Let's see a few small examples. We have 2 = 1² + 1², so we say that 2 is S2. Similarly 4 = 2² + 0² is S2. Then 2 × 4, that is to say, 8, should be S2 as well. Indeed, 8 = 2² + 2².
Another, slightly less trivial example. We've seen that 13 and 32 are both S2. Then their product, 416, should also be S2. Lo and behold, 416 = 20² + 4², so indeed it is S2.
How do we know this will always work? The simplest way, as long as you've already internalized the bit from Part 1 about absolute values, is to think about the norms of complex numbers. A norm is, quite simply, the square of the corresponding distance. (Okay yes it can also mean different things in other contexts, but for our purposes that's what a norm is.) The norm is written with double bars, so ‖3+6i‖ = 45 and ‖5-2i‖ = 29 and ‖4+3i-7j+4k‖ = 90.
One thing to notice is that if your starting numbers are whole numbers then the norm will also be a whole number. In fact, because of how we've defined lengths, the norm is just the sum of the squares of the real-number bits. So, any S2 number can be turned into a norm of a complex number: 13 can be written as ‖3+2i‖, 32 can be written as ‖4+4i‖, and 49 can be written as ‖7+0i‖.
The other thing to notice is that, since the absolute value is multiplicative, the norm is also multiplicative. That is to say, for example, ‖(3+6i) × (5-2i)‖ = ‖3+6i‖ × ‖5-2i‖. It's pretty simple to prove that this will work with any numbers you choose.
But lo, gaze upon what happens when we combine these two facts together! Consider the two S2 values 13 and 32 from before. Because of the first fact, we can write the product 13 × 32 in terms of norms: 13 × 32 = ‖3+2i‖ × ‖4+4i‖. So far so good. Then, using the second fact, we can pull the product into the norms: ‖3+2i‖ × ‖4+4i‖ = ‖(3+2i) × (4+4i)‖. Huzzah! Now, if we write out the multiplication as (3+2i) × (4+4i) = (4+20i), we can get a more natural looking norm equation: ‖3+2i‖ × ‖4+4i‖ = ‖4+20i‖ and finally, all we need to do is evaluate the norms to get our product! (3² + 2²) × (4² + 4²) = (4² + 20²)
The cool thing is that this works no matter what your starting numbers are. 218 = 13² + 7² and 292 = 16² + 6², so we can follow the chain to get 218 × 292 = ‖13+7i‖ × ‖16+6i‖ = ‖(13+7i) × (16+6i)‖ = ‖166+190i‖ = 166² + 190² and indeed you can check that both extremes are equal to 63,656. No matter which two S2 numbers you start with, if you know the squares that make them up, you can use this process to find squares that add to their product. That is to say, the product of two S2 numbers is S2.
Part Four: Why do we skip three?
Now we have all the ingredients we need for our cute little proof soup! First, let's hop to the quaternions and their norm. As you should hopefully remember, quaternions have four terms (some number of units, some number of i's, some number of j's, and some number of k's), so a quaternion norm will be a sum of four squares. For example, ‖4+3i-7j+4k‖ = 90 means 90 = 4² + 3² + 7² + 4².
Since we referred to sums of two squares as S2, let's say the sums of four squares are S4. 90 is S4 because it can be written as we did above. Similarly, 7 is S4 because 7 = 2² + 1² + 1² + 1², and 22 is S4 because 22 = 4² + 2² + 1² + 1². We are of course still allowed to use zeros; 6 = 2² + 1² + 1² + 0² is S4, as is our friend 13 = 3² + 2² + 0² + 0².
The same fact from the S2 numbers still applies here: since 7 is S4 and 6 is S4, we know that 42 (the product of 7 and 6) is S4. Indeed, after a bit of fiddling I've found that 42 = 6² + 4² + 1² + 1². I don't need to do that fiddling, however, if I happen to be able to calculate quaternions! All I need to do is follow the chain, just like before: 7 × 6 = ‖2+i+j+k‖ × ‖2+i+j‖ = ‖(2+i+j+k) × (2+i+j)‖ = ‖2+3i+5j+2k‖ = 2² + 3² + 5² + 2². This is a different solution than the one I found earlier, but that's fine! As long as there's even one solution, 42 will be S4. Using the same logic, it should be clear that the product of any two S4 numbers is an S4 number.
Now, what goes wrong with three dimensions? Well, as you might have guessed, it has to do with S3 numbers, that is, numbers which can be written as a sum of three squares. If we had any three-dimensional number system, we'd be able to use the strategy we're now familiar with to prove that any product of S3 numbers is an S3 number. This would be fine, except, well…
3 × 5 = 15.
Why is this bad? See, 3 = 1² + 1² + 1² and 5 = 2² + 1² + 0², so both 3 and 5 are S3. However, you can check without too much trouble that 15 is not S3; no matter how hard you try, you can't write 15 as a sum of three squares.
And, well, that's it. The bucket has been kicked, the nails are in the coffin. You cannot make a three-dimensional number system with the kind of nice norm that the complex numbers and quaternions have. Even if someone comes to you excitedly, claiming to have figured it out, you can just toss them through these steps: • First, ask what the basis is. Complex numbers use 1 and i; quaternions use 1, i, j, and k. Let's say they answer with p, q, and r. • Second, ask them to multiply (p+q+r) by (2p+q). • Finally, well. If their system works, the resulting number should give you three numbers whose squares add to 15. Since that can't happen, you've shown that the norm is not actually multiplicative; their system doesn't capture the geometry of three dimensions.
#math#numbers#human interaction#this took the better part of a day to write oops#although to be fair I haven't exactly been focused#Also hi Pyro! Welcome.#that silly fast food emoji post went wild#I've gotten 30 followers just from that one post#which isn't that many in objective terms but like it's 40% of my current count so#hello everyone#I might start reblogging things again now
299 notes
·
View notes
Text
happy pi day to all the wonderful math-loving radfems on here! yall motivate me to study for my calc class <3
#do NOT ask me about my math grade#it willl get better during finals trust#radblr#radical feminist community#radfemsafe#radical feminists do interact#radical feminists do touch#radical feminist safe#<3
60 notes
·
View notes
Text

turned this part of an official tiktok into a gif bc im obsessed with this
#when the adhd hits or something BHDNJSFBHNJ#it also reminds me of that one confused lady gif with all the math too DSBGHNDGDBHGJ#but genuinely that skit was super cute! love seeing the g1 and g3 iterations interacting with each other!#anyways feel free to swipe this for your own confused reactions/whatever#monster high#🦴 rattles
82 notes
·
View notes
Text
Math lovers of tumblr, what's your favourite math subject? And what's your least favourite? And: why?
40 notes
·
View notes
Text
I feel like my spirit animal is a robot. I got into a stem program when I was 6, vocaloid music slaps, BEACH BEAR (emphasis on the beach bear), the roquefort explosion 💥, HEX is my favorite fnf mod (not to mention I was there for the beginning of fnf) and I find him way too relatable 😭uhm
might make a robot variant of my sona
#robots#im legit gonna go get my sketchbook after this is posted#ermm#beep boop i guess#lmao#also i think like a robot if that makes sense#e.g. for me to learn a new kind of math i need a set of steps to follow every time#same with some forms of social interaction LMAO#rock afire explosion#rockafire explosion#showbiz pizza#beach bear#rockafire#the rock afire explosion#why am i finding robots relatable#chat am i cooked#friday night funkin#hex fnf
18 notes
·
View notes
Text

So this doesn't look anything like the last chart because I do not remember the math I used for the last one, I wasted too much time on it because I was procrastinating, and I'm not doing it again.
Anyway, we're doing great.
#the second sight#interactive fiction#lmao ignore the subtitle i didn't remove#although my math probably is bad enough to be considered fictional
21 notes
·
View notes
Text
Jason: The alphabet is somewhere between 25-27 letters.
Nick: Oh yeah, it used to be 28 but they got rid of some letters due to budget cuts.
Jason, counting on his fingers: No, yeah, it's 26.
Nick: THAT WASN'T A BIT?!?!?!?
#house of ashes#jason kolchek#nick kay#dark pictures house of ashes#incorrect house of ashes#i fear this is word for word an interaction i had with my cousin#i was nick#hoa#but also i'm shit at math
18 notes
·
View notes
Text
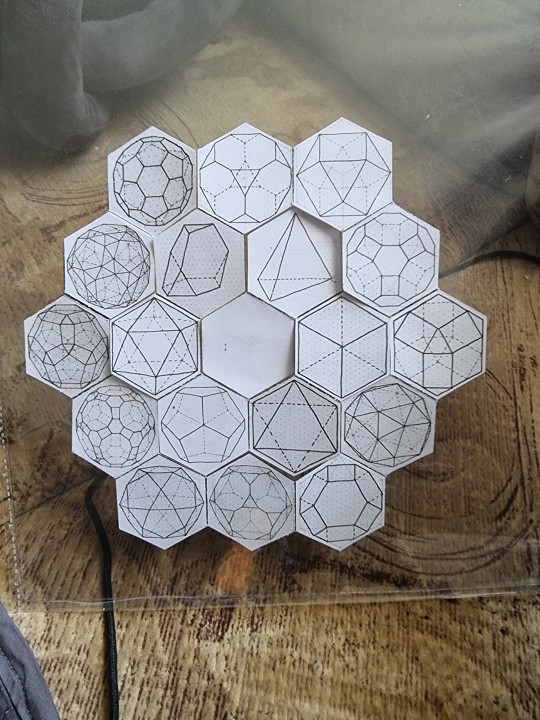

Front: 5 platonic solids and the 13 Archimedean solids
Back: Root-like structures, depicting some relationships between these 18 solids.
Both simultaneously (Holding it against light source):

#platonic solids#archimedean solids#geometry#geometric#solids#shapes#shape soup#polyhedra#polyhedron#shape#hexagon#hexagonal#hexagons#hexagonal book#interactive art#knottys math#math art
81 notes
·
View notes